We want to compute the integral \(I=\int_{\frac{-T}{2}}^{\frac{T}{2}}\int_{\frac{-T}{2}}^{\frac{T}{2}} dt' dt f(t'-t)\), which appears frequently in Fouirer transforms. For instance, the proof of Wiener-Khinchin theorem requires evaluation of such an integral.
The argument of \(f\) begs for a change of coordinates:
\[\begin{eqnarray} u=t'-t, \quad \text{and} \quad v=t+t' \tag{1}, \end{eqnarray}\] and the associated inverse transform reads: \[\begin{eqnarray} t'=\frac{u+v}{2}, \quad \text{and} \quad t'=\frac{v-u}{2}. \tag{2} \end{eqnarray}\]
This transformation will rotate and scale the integration domain as shown in Fig. 1.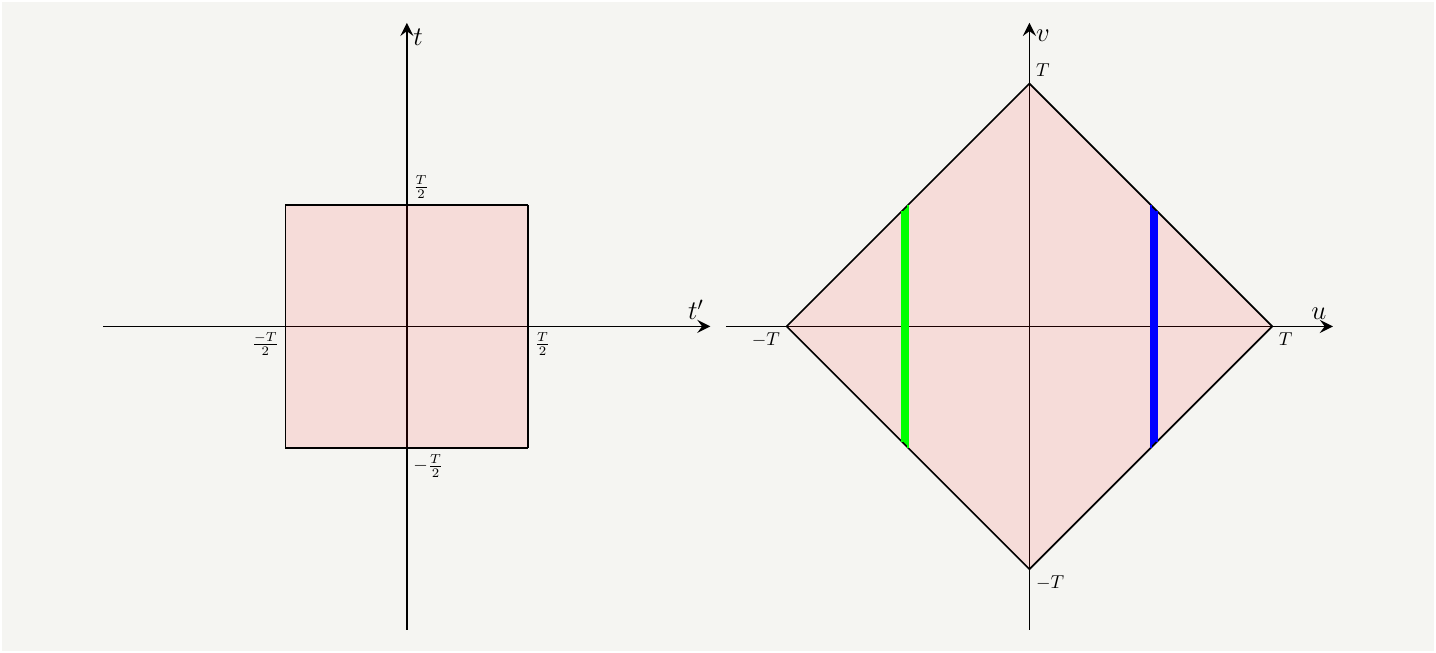
Figure 1: The integration domain in the \(t-t'\) domain (left) and \(u-v\) domain(right). Since there is no \(v\) dependence, \(v\) integration gives the height of the green and blue slices.
The equation of the top boundary on the right can be written as \(v=T-u\), and on the left as $ v= T+u$. We can actually combine them as \(v=T-|u|\). We can do the same analysis for the lower boundaries to see that the height of the slices at a given \(u\) is \(2(T-|u|)\). This will help us easily integrate \(v\) out as follows: \[\begin{eqnarray} I&=&\int_{\frac{-T}{2}}^{\frac{T}{2}}\int_{\frac{-T}{2}}^{\frac{T}{2}} dt' dt f(t'-t) =\iint_{S_{u,v}}\left|\frac{\partial(t,t')}{\partial(u,v)}\right| dv du f(u)\nonumber\\ &=&\int_{-T}^T 2(T-|u|) \times\frac{1}{2} dv du f(u)=\int_{-T}^T du f(u)(T-|u|) \tag{3}, \end{eqnarray}\] where \(\left|\frac{\partial(t,t')}{\partial(u,v)}\right|=\frac{1}{2}\) is the determinant of the Jacobian matrix associated with the transformation in Eq. (2).